SNOWFLAKES,MANDALA DESIGNS AND ROTATIONAL SYMMETRY: THE GEOMETRY OF ART

paper snowflakes by middle school students

ROTATIONAL SYMMETRY EXPLAINED from Rachel Wintemberg on Vimeo.

IF YOU SUPERIMPOSE TWO IDENTICAL IMAGES AND ROTATE THE TOP DESIGN AROUND A SINGLE POINT, HOW MANY TIMES WILL THE TWO DESIGNS MATCH UP EXACTLY BEFORE MAKING A COMPLETE CIRCLE?

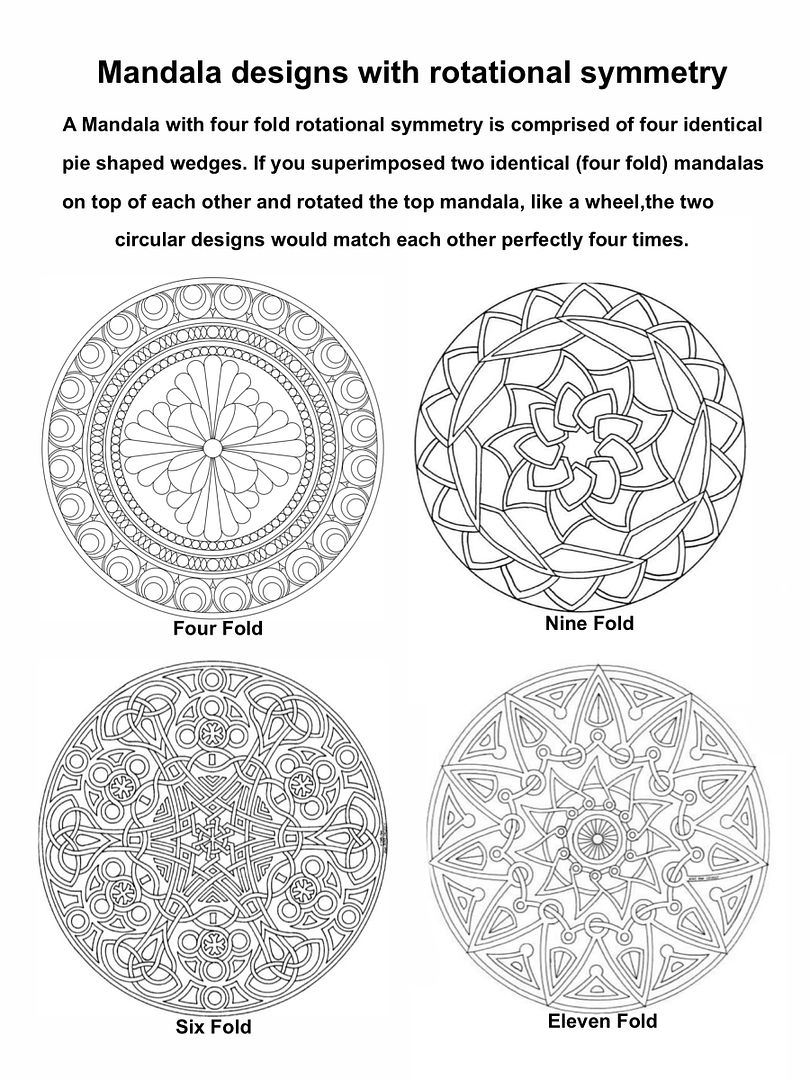
This mandala wheel has twelve fold symmetry, because the top wheel matches the bottom wheel twelve times. In other words, this design is comprised of twelve identical slices around a central point. Each of these slices is 30 degrees of the whole (360 degree) circle (30x12=360).

Snowflakes have six fold rotational symmetry

A finished paper cut out snowflake

Here are complete printable step-by-step directions for creating your own paper snowflake with six fold symmetry:

Help the Salvation Army! Create a snowflake online by clicking here.

Can you increase your
 visual-spatial intelligence while making paper snowflakes?

Yes. Here is how:

After you have followed steps one through three, open up the shape and take a look at it.  It should be a hexagon (six sided shape).

Now, carefully fold it up again and cut one small triangular scrap from your paper. Do not unfold it yet.

Try to guess what the snowflake will look like unfolded. Draw a small sketch of a hexagon and draw where you think the holes should go. Now unfold your paper to check your answer.

Once you have mastered this, try it with two holes, then three, then four.

What happens if you cut a shape from the point of the cone?

What happens when you cut from the open side (base) of the cone?

What happens when you cut out a curved shape? 
How about a zig-zag shape?

This mental exercise will actually increase your visual spatial thinking ability and help you to perform better on intelligence tests.

Click here to see a sample of a paper folding spatial ability test.

Snowflakes and math: The koch snowflake

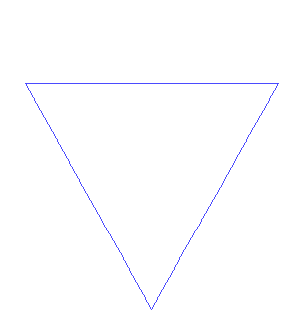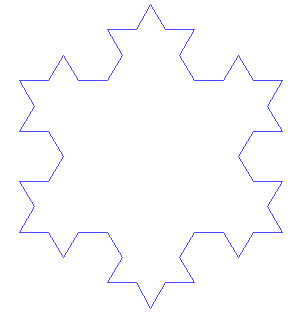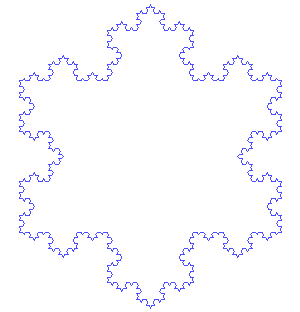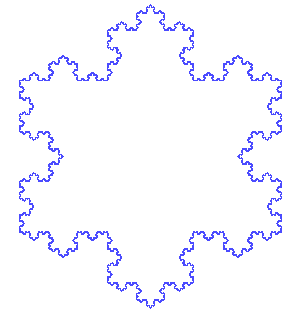The Koch Snowflake is an infinite, repeating fractal pattern.
To learn what a fractal is, click here
For step-by-step directions to create your own Koch Snowflake click here

Click on the link below to watch a real snowflake crystal grow:

http://thekidshouldseethis.com/post/77698700764

MAKING CONNECTIONS

http://www.its.caltech.edu/~atomic/snowcrystals/class/class.htm

WHAT DO SNOWFLAKES, GEOMETRY AND TRADITIONAL MANDALA DESIGNS HAVE IN COMMON?

Use this website to create exquisite symmetrical mandala designs:  http://weavesilk.com/

Watch this video to learn about rotational symmetry and the geometry of snowflakes, flowers and mandala designs                                                                           

INTO THE EYE OF THE MANDALA, ROTATIONAL SYMMETRY from Rachel Wintemberg on Vimeo.

To learn more about rotational symmetry, click here

Watch the video below to learn how to create your own Mandala drawing 

ARCHAEOLOGISTS HAVE DISCOVERED ANCIENT DESIGNS WITH ROTATIONAL SYMMETRY THROUGHOUT THE WORLD.

Rotational symmetry designs in Pre-Colombian Native American Pueblo Pottery

Rose Window, Chartres Cathedral, France

 Rotational symmetry is found throughout the Pre-Colombian art of the Americas but until the first European explorers came to the New World none of the Native Americans had ever seen a wheel and axle.

 How could Native Americans have created designs based on a rotating wheel and yet never invent any simple machines using the wheel? Living on top of a Mesa, pueblo potters would have had no use for wheeled carts.

Ancient pueblo potters built their vessels using ropes of clay, called coils. They pressed the clay into woven baskets or into the bottom of a broken ceramic pot in order to form their vessels. They continuously rotated their work in front of them in order to press the clay evenly. The spiral pattern of the clay coils or woven baskets inspired these early artisans to use rotational symmetry in their designs. 

 Why do you think rotational symmetry existed in so many prehistoric cultures throughout the world? 

Many cultures consider a wheel design with rotational symmetry (or Mandala) to be a spiritual or meditative symbol. The following excerpt on Mandalas is from Wikipedia:  

Mandala (Sanskrit: मण्डल Maṇḍala, 'circle') is a spiritual and ritual symbol in Hinduism and Buddhism, representing the Universe.^[1] The basic form of most mandalas is a square with four gates containing a circle with a center point. Each gate is in the general shape of a T.^[2][3] Mandalas often exhibit radial balance.^[4]

The term is of Hindu origin. It appears in the Rig Veda as the name of the sections of the work, but is also used in other Indian religions, particularly Buddhism.

In various spiritual traditions, mandalas may be employed for focusing attention of aspirants and adepts, as a spiritual teaching tool, for establishing a sacred space, and as an aid to meditation and trance induction.

In common use, mandala has become a generic term for any plan, chart or geometric pattern that represents the cosmos metaphysically or symbolically; a microcosm of the universe from an enlightened perspective; i.e., that of the principal deity

During the Middle Ages, European architects built Gothic Cathedrals with exquisite rose windows. Parishioners gazed up at the jewel like ethereal light streaming through the stained glass and contemplated the divine. To learn more about rose windows, click here.

To learn more about Medieval Gothic cathedrals, watch this excellent film by David Macaulay:

To learn how to design your own Medieval Gothic rose window, click here.

Click here to view some amazing stained glass windowsClick on the link below to find another collection of stained glass rose windows from all over the world:

http://www.flickr.com/groups/90427824@N00/

Rotational symmetry appears in unexpected places, like the unfolding petals of a flower or an exploding firework. Keep your eyes open and you will find it everywhere. 

ROTATIONAL SYMMETRY, PRINTABLE WORKSHEETS